This past week, Ciprian Manolescu posted a preprint on ArXiv proving (allegedly- I haven’t read the paper beyond the introduction) that the Triangulation Conjecture is false.
-equivariant Seiberg-Witten Floer homology and the Triangulation Conjecture.
This is big news. I feel it’s the last nail in the coffin of the Hauptvermutung. I’d like to tell you a little bit about the conjecture, and about Manolescu’s strategy, and what it has to do with low dimensional topology.
What is an  -manifold?
-manifold?
“That’s a silly question,” someone might say. “It’s a (bla bla bla) space that is locally homeomorphic to ”.
Ah, but homeomorphic via what kind of map? A continuous map? A smooth map? A piecewise linear map? In two dimensions it makes no difference. But in higher dimensions, the concept of an -manifold turns out to be more subtle than the
case might lead you to believe.
For Poincaré in 1895, a `three manifold’ starts out as a quotient of by actions of certain groups with a cube as fundamental region. Later in Analysis Situs, he presents a more general notion of a manifold by identifying faces of polyhedra. Poincaré’s manifolds were supposed to be differentiable, but his constructions of them are PL. It seems that Poincaré considered that there was no difference. Obviously all corners can be smoothed and all smooth structures can be approximated by broken linear structures, and (although this notion came later) obviously topological manifolds can all be triangulated. Obvious, but false. The shocking truth is that smooth, PL, and topological categories diverge sharply, even if we restrict ourselves to thinking about individual manifolds and not maps between manifolds.
The following explanation is distilled from Ranicki’s excellent survey On the Hauptvermutung.
A triangulation of a topological space is a simplicial complex
together with a homeomorphism
from
the polyhedron of
to
. A space is triangulable if it admits a triangulation. In 1908, Steinitz and (independently) Tietze formulated the Main Conjecture, or Hauptvermutung, which states that triangulations of homeomorphic spaces are combinatorially equivalent i.e. become isomorphic after subdivision. In 1961, Milnor shocked the mathematical world by finding a counterexample. But his counterexamples were not manifolds- and as geometric topologists, we’re only supposed to care about manifolds, right? (I really needed sarcastices for that last sentence). So is the Hauptvermutung at least true for manifolds? Closely related, we have the:
Combinatorial Triangulation Conjecture
Every compact topological manifold can be triangulated by a PL manifold.
In dimension two the conjecture holds by 1925 work of Radó, and in dimension three by work of Moise. But (prepare to be shocked again) it’s false in dimensions by work of Kirby and Seibenmann, and in dimension
by work of Freedman.
Poor Hauptvermutung! Can’t it at least be just a little bit right?
Let’s try again… Maybe to be homeomorphic to a PL manifold is too much to ask. Recall that the link of a simplex σ is the complex of all simplices at `distance one’ from σ (i.e. separated by one edge from σ). A PL manifold has the property that the link of each simplex of its triangulation is a sphere. Maybe this is too much to ask. After all, there are plenty of not-terribly-pathological spaces which don’t satisfy this property, such as the double suspension of any non-trivial homology sphere (such as the Poincaré sphere), by Cannon and Edwards’s Double Suspension Theorem. So let’s weaken the Combinatorial Triangulation Conjecture:
Triangulation Conjecture
Every compact topological manifold can be triangulated by a locally finite simplicial complex.
Unfortunately even this fails in dimension four, with Freedman’s -manifold providing a counterexample… but maybe dimension four is pathological. Maybe it holds in dimension greater than five.
For all the seeming simplicity of the statement, there’s another surprise in store- even though it’s about manifolds in dimension , it actually reduces to a problem in low dimensional topology of smooth three manifolds. It’s a problem about holomogy cobordism of homology spheres, so let’s briefly review that story.
By the way, the paper I first learnt this story from (and which made a deep impression on me) is:
Ruberman, D., & Saveliev, N. (2005). Casson-type invariants in dimension four. Geometry and topology of manifolds, Fields Inst. Commun, 47, 281-306.
Recall that an integral homology sphere is a closed oriented -manifold
with
. There are plenty of these, the most famous perhaps being the Poincaré homology sphere. A homology cobordism between homology spheres
and
is a compact oriented
-manifold
with boundary
such that the inclusions
induce isomorphisms
for
. Homology cobordism is an equivalence relation, and the set of equivalence classes with operation the connect sum forms a group
.
Next let’s recall the Rokhlin Invariant. Every integral homology sphere is the boundary of a compact spin -manifold
, and the Rokhlin invariant is an eighth of the signature of
modulo two. It is invariant under homology cobordism, and so defines a homomorphism
.
Alright now! Galewski and Stern, and independently Matsumoto, reduced the Triangulation Conjecture to the following:
Equivalent Statement to the Triangulation Conjecture
There exists a homology-sphere
with Rokhlin invariant one which is of order two in
.
So the goal becomes to understand torsion in (at least for Rokhlin invariant one homology spheres). It turns out not to be easy, probably because it’s a statement about the smooth category, and so many of our techniques are secretly PL. Until the 1980’s, all that was known about
was that
is an epimorphism. Then, using equivariant gauge theory, Fintushel and Stern showed that
has many elements of infinite order, and Furuta showed that it is infinitely generated. But does it have
-torsion? How would you even approach a question like that?
Manolescu’s answer is to construct a Seiberg–Witten Floer Homology with all of the symmetries that the Seiberg-Witten equations have in the situation at hand. Namely, because the bounded four manifold has a spin structure, the Seiberg–Witten equations turn out to have a symmetry group known as
. Manolescu has experience with equivariant Seiberg–Witten theories, and he constructs a weapon which (to my untrained eye) looks big enough and strong enough for the task. There’s probably a lot more that this Floer homology can do, and one can now expect vigourous progress in its study.
All of this leaves the Hauptvermutung pretty much as dead as dead can be. Topological manifolds in dimension greater than three just can’t be triangulated in general… And we’re going to have to learn to live with it.
Edit: As pointed out in the comments, by Galewski-Stern dimension 5 counterexamples must be non-orientable (which is quite striking!), but in dimension 6 and above, there are orientable counterexamples as well.
Executive summary for the casual mathematical tourist
Given a lego set whose blocks are triangles (i.e. simplices), there are many shapes (i.e. compact topological manifolds) you could build. But could you build all of them?
In dimension 1 you obviously could (a circle can be cut into line segments), in dimension 2 you obviously could (any compact surface can be sliced up into triangles, and you take those to be the blocks). It turns out that you can in dimension 3 as well (intuitively unsurprising, but difficult to prove), but surprisingly you cannot in dimension 4. But we all know that dimension 4, being the dimension in which we live (space plus time) is a bit crazy- what about in dimension 5 and higher? Galewski and Stern showed that you can triangulate any shape (shape= compact topological manifold) in dimension 5 and up (in just about the weakest sense that a decomposition into triangles has any right to call itself a triangulation) if and only if you can triangulate a specific 5-dimensional shape called the Galewski-Stern manifold. Manolescu’s preprint proves you cannot, showing us again that there are more things in heaven and earth, Horacio, than are dreamt of in your (triangulated) philosophy.
Actually, Professor Moskovich, it is not as grim as you point out. As mentioned in Galewski and Stern’s original paper, “A Universal 5-Manifold With Respect to Simplicial Triangulations” (It is published in the book Geometric Topology edited by James Cecil Cantrell on the Georgia Topology Conference 1977), open and/or orientable 5 manifolds CAN always be simplicailly triangulated. The obstruction only occurs for compact non-orientable manifolds.
Comment by Mayer A. Landau — March 17, 2013 @ 12:10 am |
Thank you for the comment! I hadn’t realized this. It makes the disproof of the Triangulation Conjecture all the more striking!
Comment by dmoskovich — March 17, 2013 @ 1:29 am |
There is also a little discussion about this n the book History of Topology edited by Ioan Mackenzie James. See page 499 of the chapter “A Short History of Triangulation and Related Matters” written by N.H. Kuiper.
Comment by Mayer A. Landau — March 17, 2013 @ 6:44 pm
Yes, it is striking. I mean, you could take the orientable double cover and you know that’s simplicially triangulable. But then, somehow, when you descend to the quotient, the triangulation falls apart. It’s almost as if the orientable double cover is at the cusp of what is possible to simplicially triangulate, and then you add a feather and the camel’s back breaks.
Comment by Mayer A. Landau — March 23, 2013 @ 2:36 am
Mayer, are you saying that all known counterexamples are non-orientable, or that it is known that any counterexample must be non-orientable?
Comment by Henry Wilton — April 11, 2013 @ 5:19 am
Hi Henry,
in answer to your question, I will quote from the original Galewski-Stern paper. To wit,
“In this paper we give a geometric construction of a closed non-orientable topological 5-manifold N^5 with the property that N^5 has a simplicial triangulation if and only if every compact topological n-manifold M^n, n greater than or equal to 6 (n greater than or equal to 5 if boundary of M is simplicially triangulated) has a simplicial triangulation. Note that Siebenmann’s Theorem B of [Siebenmann, L., Are non-triangulable manifolds triangulable?, pp. 77-84 in Topology of Manifolds ed. by J.C. Cantrell and C.E. Ewards, Markham Chicago, 1970.] and the double suspension theorem ([Cannon, J.W., Shrinking cell-like decompositions of manifolds; Codimension three, Annals of Mathematics
Second Series, Vol. 110, No. 1 (Jul., 1979), pp. 83-112], [Edwards, R. D., The double suspension of a certain homology 3-sphere is S^5, Notices A.M.S., 22 (1975), A 334. Abstract #75 T-G 33. ]) show that all open or oriented closed 5-manifolds can be simplicially triangulated.”
So, from that paragraph, a counterexample in dimension 5 must be non-orientable and compact.
Could a manifold of dimension greater than 5 be orientable and compact and still not be simplicially triangulable? I would guess not because you build up the higher dimensional counterexamples by taking a Cartesian product with a non-orientable (non-simplicially triangulable) 5-manifold, and the Cartesian product with a non-orientable manifold is non-orientable. But, I hope professor Manolescu is reading this and can tell me if I’m right or wrong here.
Comment by Mayer A. Landau — April 12, 2013 @ 12:36 am
Thanks for that, Mayer. If all non-triangulable manifolds are non-orientable (as it seems is the case in dimension 5) then I would take issue with Daniel’s assertion that ‘the Hauptvermutung [is] pretty much as dead as dead can be’. Orientability is a fairly mild assumption!
Comment by Henry Wilton — April 12, 2013 @ 8:08 am
[silly comment of mine deleted]
Comment by dmoskovich — April 13, 2013 @ 8:37 am
Hi Dr. Moskovich,
as Professor Stern pointed out in comment 3 below, you can have non-simplicially triangulable ORIENTABLE compact manifolds in dimension greater than or equal to 6.
Comment by Mayer A. Landau — April 13, 2013 @ 1:08 pm
I’ve deleted the entire “Peter” thread.
Comment by Ryan Budney — April 10, 2013 @ 7:05 pm |
Take a non-orientable circle bundle over the Galewski-Stern universal 5-manifold example. This is an orientable 6-manifold and their work shows that it is not a simplicial complex (and is also universal – i.e. ). Any cartesian product of this with a triangulable manifold will also not be triangulable.
). Any cartesian product of this with a triangulable manifold will also not be triangulable.
Comment by Ronald J. Stern — April 12, 2013 @ 1:25 pm |
Thank you Professor Stern for that clarification.
Comment by Mayer A. Landau — April 12, 2013 @ 4:52 pm |
After reading this thread, perhaps some clarification is necessary. The original work of Galewski-Stern (Classification of simplicial triangulations of topological manifolds. Ann. of Math. (2) 111 (1980), no. 1, 1–34) gave a complete theory as to when a topological manifold M (of dim >4) is a simplicial complex, and if it is, how many distinct such triangulations there are up to concordance. In particular there is a short exact sequence (
( is the Kervaire-Milnor-Rochlin invariant). Let
is the Kervaire-Milnor-Rochlin invariant). Let 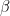 be the Bockstein associated with this short exact coefficient sequence. Then a manifold M is a simplicial complex iff
be the Bockstein associated with this short exact coefficient sequence. Then a manifold M is a simplicial complex iff 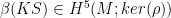 vanishes . If
vanishes . If  there are
there are  triangulations up to concordance.
triangulations up to concordance.
The existence of the “universal” 5-manifold is a cute corollary and construction, for it follows from all of this that if there is an n-manifold M with that is triangulable, then all n-manifolds (n>4) are triangulable. (Here
that is triangulable, then all n-manifolds (n>4) are triangulable. (Here  is the first Steenrod square, i.e. the Bockstein in the coefficient exact sequence
is the first Steenrod square, i.e. the Bockstein in the coefficient exact sequence  There are generalizations of this in the original Annals paper that take into account that while there may not be an element of Rochlin invariant one of order 2, there might be elements of other even order. The statement that does not require anything about the existence of a certain homology 3-spheres is that if the integral Bockstein of KS vanishes, then the manifold is a simplicial complex. Again this is all in the original 1976 Annals paper. Hope this helps.
There are generalizations of this in the original Annals paper that take into account that while there may not be an element of Rochlin invariant one of order 2, there might be elements of other even order. The statement that does not require anything about the existence of a certain homology 3-spheres is that if the integral Bockstein of KS vanishes, then the manifold is a simplicial complex. Again this is all in the original 1976 Annals paper. Hope this helps.
Comment by Ronald J. Stern — April 12, 2013 @ 5:32 pm |
Thank you very much for this explanation!
Comment by dmoskovich — April 13, 2013 @ 8:24 am |
[…] hopes of being able to divide certain types of surfaces into triangles in a specific way. Blog post on the topic, with an interesting comments discussion (via Dave Richeson on […]
Pingback by The Aperiodical | Not mentioned on The Aperiodical this month, March/April — April 15, 2013 @ 4:14 am |
A preprint was posted on the ArXiv today titled “Asphereical manifolds that cannot be triangulated”, by Davis, Fowler, and Lafont. They prove that the simplicial triangulation conjecture fails in dimension six or greater using different techniques then Manolescu. In the second to last paragraph they ask the question of whether there exists an orientable manifold that is not simplicially triangulable. They answer by giving the same example as Stern did above, although they do not cite his paper.
Comment by Mayer A. Landau — April 15, 2013 @ 10:02 pm |
It is not accurate to say that Davis-Fowler-Lafont use different techniques than Manolescu. In fact, they do not give an independent proof of Manolescu’s theorem; rather, they assume it and then show that using it together with some other techniques one can produce non-triangulatable manifolds which are also aspherical.
Comment by Andy Putman — April 15, 2013 @ 10:45 pm |
Hi Professor Putnam,
I guess I missed that. Where in their paper do they assume Manolescu’s theorem in their proof?
Comment by Mayer A. Landau — April 16, 2013 @ 9:14 am
The claim that the “Galewski-Stern 5-manifold” is not triangulable is where they are using Manolescu.
Bravo! The plot thickens! There are aspherical non-triangulable manifolds in dimension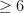 .
.
Comment by dmoskovich — April 16, 2013 @ 9:31 am |
Hi Dr. Moskovich. and
and  are both nonzero” (page 7, 1st paragraph) to draw the conclusion that
are both nonzero” (page 7, 1st paragraph) to draw the conclusion that  is not simplicially triangulable.
is not simplicially triangulable.
I think Manolescu is implicitly implied after the statement “
Can anyone tell me if I’m correct/incorrect here?
Comment by Mayer A. Landau — April 16, 2013 @ 10:28 am
Yes, this is correct. However, there is an incorrect statement in the DFL paper (already pointed out to DFL). They assumed that the Manolescu map from to the integers is a homomorphism. Manolescu did not prove this (yet – see the last paragraph of the Manolescu manuscript for ideas how to proceed). Thus there is the (unlikely) possibility that there is a Rochlin invariant one homology 3-sphere with even order
to the integers is a homomorphism. Manolescu did not prove this (yet – see the last paragraph of the Manolescu manuscript for ideas how to proceed). Thus there is the (unlikely) possibility that there is a Rochlin invariant one homology 3-sphere with even order  ). So DFL need to check that the first Steenrod square (not the integral Bockstein β) of
). So DFL need to check that the first Steenrod square (not the integral Bockstein β) of  is non-trivial. Then the work of Galewski-Stern together with Manolescu imply that
is non-trivial. Then the work of Galewski-Stern together with Manolescu imply that  is not a simplicial complex. (For GS point out that if such a manifold were a simplicial complex, then there would be a Rochlin invariant one homology 3-sphere with order 2, but Manolescu proves there is no such homology 3-sphere).
is not a simplicial complex. (For GS point out that if such a manifold were a simplicial complex, then there would be a Rochlin invariant one homology 3-sphere with order 2, but Manolescu proves there is no such homology 3-sphere).
Comment by Ronald J. Stern — April 16, 2013 @ 11:08 am |
[…] that, according to a 2013 paper, some manifolds are not even homeomorphic to any polyhedron. See https://ldtopology.wordpress.com/2013/03/16/manolescu-refutes-the-triangulation-conjecture/ for a brief survey of these results. ____________________________________ By "manifold" […]
Pingback by Triangulation of circle and disk in R2 | Physics Forums - The Fusion of Science and Community — April 12, 2016 @ 7:36 pm |